Dr. Cooper's comments

 

Red text - Beamelak Tafesse

 

Partial molar gibbs energy :  The concept of partial molar quantities can be extended to any extensive state function. For a substance in a mixture, the chemical potential is defined as the partial molar gibbs energy.

 

      

 

 (Equation 1)

 FIGURE 1

 

 

For a pure substance, the Gibbs energy  

 

(Equation 2)

 

= using the same arguments for the derivation of partial molar volume, we can derive the total gibbs energy iof a binary mixture that is given by 

 

 

(Equation 3)

 

From equation 3 the formula explains that the gibbs energy of a binary mixture is equal to the component in a mixture of A added to that of B assuming that pressure and temprature remains constant.

 

Fundamental Equation of chemical Thermodynamics: In general the gibbs energy depends upon the composition, temprature and pressure. when any of these variables alter, G may change. so for a system that has components A, B, etc, it is possible to rewrite the general equation,

 

dG=VdP-SdT(which is a general result that was derived here) as follows

 

which is called the fundamental equation of chemical thermodynamics.

 

(Equation 4) 

 

The equation then simplifies 

(at constant pressure and temprature)

 

(Equation 5)

under this condition dG = dW, where the n represents the work is not expansion work. Therefore,

(at constant temprature and presure)

 

(Equation 6)

 

The idea that the changing composition of a system can do work should be familiar- this is what happens in an electrochemical cell. where the two halves of the chemical reaction are separated in space at the two electrodes and the changing composition results in the motion of electrons through a circuit, which can be used to do electrical work.

It is possible to use the relationships between G and H, and G and U, to generate the relations 

 ,   

 

Not only can we ralate partial chemical energy to gibbs energy, it can also be related to other thermodynamics.

 

G = H - TS = U + PV - TS

U - PV + TS + G... by looking at the infinitismal change...

 

  

At constant temprature and volume, dV and dS are going to be zero. Therefore the equation becomes

 

(Equation 7)

 

 

Gibbs - Duhem Equation: In thermodynamics, the gibbs - duhem equation describes the relationship between changes in chemical potential for components in a thermodynamical system.

 

 

(Equation 8)

 

...at constant temprature and pressure,

 (at constant pressure an temprature)

(Equation 9) 

 

, if we rearrange this equation, , here chemical potential of B cannot change without chemical potential of A.

 

Molarity ; which is represented by the symbol C, is the amount of solute divided by the volume of the solution. molarity has a units of mole per decimeter cubed or moles per liter.

Molality : which is represented by the letter b, is the amount of solute divided by the mass of solvents. The unit of molality is mole per kilogram.

 

Using Gibbs - Duhem Equation:

 

The experimental values of partial molar volume of potassium sulfate at 298k are found to fit the expression that is described by,

 

                                                            

                                                            

                                                             molality of potassium sulfate 

 

we know from the above equation that gibbs energy at constant pressure and volume is    

                                        Therefore,    . Here the partial molar volume to A has to depend upon B.

Thereby, integrating both sides,            

                                                             

                                                            

                                                                          

                                                                           (Equation 10)

 

We also know that the partial volume of potassium responds to

 

                                                            

                                                            

                                                            

 

From equation 10 above we know that        

 

                                                         

                                                         

                                                          will be used to calculate the partial volume of potassium sulfate.

 

 

  

                                                          where x is the molality, A= water and B= potassium sulfate.

FIGURE 2

 

 

Tram Tran

 

THERMODYNAMICS OF MIXING

 The mixing two gases a spontaneous process and implies Gibbs free energy change during spotaneous process and leads to a delta G that is negative.  Figure  1  is general model of a Gibbs free energy change when gases start mixing.  If Temperature and pressure remain unchanged during the mixing process,  we can define the fnial pressure simply by knowing the  partial molar quantities.

 

 

 

Figure 1: We can find total pressure by knowing partial pressures of A and B and also the temperature (T)

---------------------

 

equation 11

 

In equation 11 we see that total pressure equals a sum of all the partial pressures.

----------------------

 

Gm = Gm' +RTln(P/P')

μ = μ' + RTln(P/P')       

μ = μ' + RTlnP          equation 12 (It would be much easier to follow if you used the equation editor for your equations)

 

In equation 12 we see that we can define the gibbs free energy at equilibrium simply by know pressure value.

 

----------------------

 

μ = μ' + RTlnp

Gi = nA(μA) + nB(μB)

Gi = nA((μ'a)+ RTlnp) + nB(((μ'b)+ RTlnp)

Gf = nA(μ'a+ RTln(pA)) + nB(((μ'b)+ RTlnpB)

ΔmixG= Gf - Gi = nARTln(pA/p) + nBRTln(pB/p)     equation 13

 

 

In equation 13 above, final formula states that final mixture has  total change gibbs free energy related  initial and final states.  It can be seen that both Gf and Gi are defined by molar quantities and initial free energy states, the terms nA and uA .  Plugging  formula u= u +RTln(p) for each Ux term allows us to find the initial gibbs free energy state.  By plug in similar formula where we use  partial pressures each individual component in for  “P” variable, we can find  final gibbs free energy state and find total free energy change that occur during the mixing.

 

 

------------------

mixG = nARTln(pA/p) + nBRTln(pB/p)

(pA/p) = xA (pB/p) = xB

ΔmixG = nARTln(xA) + nBRTln(xB)

xBn = nAxBn = nB

ΔmixG = nRT(xAln xA + xBln xB)

ΔmixG < 0                                        equation 14

 

In equations 14 we have derive alternative formula for finding  change in gibbs free energy where we replaced the Px/p variable with Xj.  Above Xj refers to either Xa or Xb; note also that if spontaneous mixing it must have a value below 0.

---------------

 

Figure 2

 

-----

(dG/dT)p,n = -S

ΔmixS = -(ΔmixG/dT)

-(d/dT)nRT(xAln xA + xBln xB) = -nR(xAln xA + xBln xB)

ΔmixS = -nR(xAln xA + xBln xB)                         equation 15

 

 

In equation(s)  15 above, we see we define change in entropy for mixing process simply know partial pressures of a and b,  relevant terms in this equation with regard to the partial pressures are the Xj terms.  Note that entropy usually defined with temperature in mind but here we have managed to get rid of all “T” terms because they cancel in the third line. 

 

-------

 

 

ΔmixG = nRT(xAln xA + xBln xB)

ΔmixS = -nR(xAln xA + xBln xB)

ΔG = ΔH - TΔS

nRT(xAln xA + xBln xB) = ΔH - T(-nR(xAln xA + xBln xB))

nRT(xAln xA + xBln xB) = ΔH + nRT(xAln xA + xBln xB)

ΔH = 0(constant p and T)                                   equation 16

 

Here in equations 16 we have summarized the defnitiions we have established into general rule for chemical thermodynamics relate entropy, enthalpy, and gibbs free energy.  Note that we have substituted our new definitions for delta G and delta S into main equation and now we find can the change in enthalpy if we know temperature and also delta G and delta S values.

 

 

<--Blue Text by Christopher Van Dolson-->

 

Ideal Solutions

 

 

The definition of an ideal solution is a solution where they enthalpy of the solution is equal to zero.  Also, the closer to zero the enthalpy, it is to be considered more “ideal” the solution is.  An ideal solution is also referenced as an “ideal mixture.” In order to discuss the equilibrium properties of a liquid mixture we first need to know and understand how Gibbs energy of liquids varies with composition.  We can use the fact that, at equilibrium, the chemical potential of a substance as a vapor must be equal to its chemical potential in the liquid form.  Figure 3 shows the equilibrium between two substances having to equal each other in its chemical potential.

 

 

 

Figure 3

 

 

 

Since the Chemical potential of a vapor equals the chemical potential of a liquid the following equation in equation 17 is true:

 

     *denotes a pure substance

 

Equation 17

 

 If there happens to be another substance added to the pure liquid, then the chemical potential of A will in turn change also.  Equation 18 shows the new equation:

 

 

Equation 18

 

 Mathematically, the following equation 19 shows how to derive an ideal solution where from above  is the pure substance:

 

 

 

 

 

 

Equation 19

 

 

A French chemist known as Francois-Marie Raoult stated that the vapor pressure of an ideal solution depends on the vapor pressure of each one of the chemical components and the mole fraction of the components that are present in the current solution.  This is known as Raoult’s Law and mathematically stated in equation 20:

 

 

Equation 20

 

 

Where the   is the vapor pressure and  is the mole fraction.  Figure 4 shows Raoult’s Law on a standard ideal solution.  While figure 5 shows that Raoult found that mixtures of closely related liquids follow equation Benzene and methylbenzene behave ideally:

 

                    

Figure 4                                                                       Figure 5

 

 

Again mathematically speaking when using Raoult’s Law in equation 20 we can use that for the ideal solution equation for liquids which is shown in equation 21:

 

 

--> Rauolt's Law

 

 

Equation 21

 

Raoult’s Law can also be used when finding non-ideal solutions or non-ideal mixtures by adding a fugacity coefficient and an activity coefficient, but for the purpose to this class is to introduce the notion of a non-ideal gas, however, Raoult’s Law can be mathematically derived to help solve for non-ideal solutions.  The non-ideal solution is a little more complicated than the ideal solution.  The following figure ## shows the graph of a non-ideal solution.  The dotted lines are Raoult’s Law, while the curved lines are the actual data provided.  In figure 6 where Raoult’s Law was introduced the straight lines are the data provided for an ideal solution.

 

Figure 6

 

 

Another notion or a subset of ideal solutions is the ideal-dilute solutions.  Figure 7 shows the new notion.

 

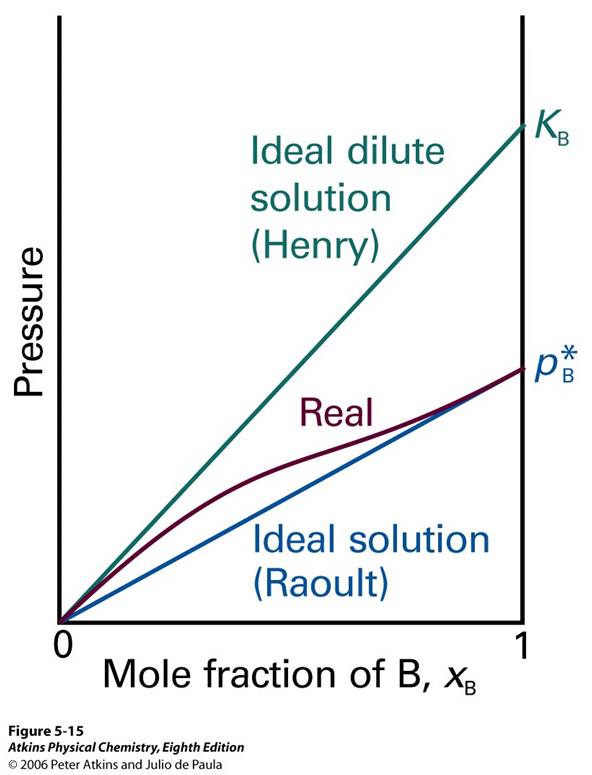
Figure 7

 

The ideal-dilute solution adds a new concept of the Henry.  For real solutions at low concentrations, although the vapor pressure of the solute is proportional to its mole fraction, the constant of proportionality is not the vapor pressure of the pure substance.  Even if there are strong deviations from ideal behavior, Raoult’s Law is obeyed increasingly closely for the component in excess as it approaches purity.  This can be reached by Henry’s Law which equaiton 22 shows the equation, where  is the partial pressure,  is the mole fraction, and is the constant of pressure divided by concentration.

 

 

Equation 22

 

  In essence mixtures for which the solute obeys Henry’s Law and the solvent obeys Raoult’s Law are called ideal-dilute solutions.